[ 12/31/2022 ] Labels: 07.Basic Study4
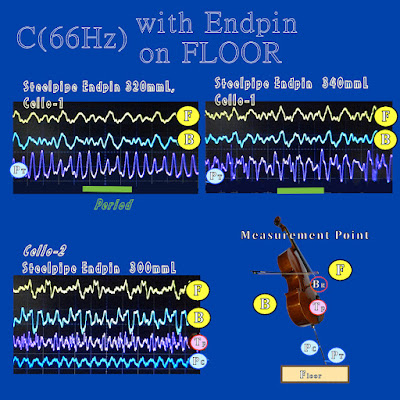
The lowest tone of cello is C(66Hz, WL=5.2m, Period=15.2mS). Open A is 221Hz, WL=1.5m, Period=4.5mS.
Careful study for a pizzicato on cello perceives us something new founding.
All pizzicatos seem having a special 4mS-period/time at their beginning, the details of 4mS-period are unidentified, we can see it at the forefront of all pizzicato.
'4mS' is presumed as an almost enough/minimum time to go and come back for the sound travels in the cello body, including both a high pressure and a low pressure.
After the short '4mS', regular periods/cycles of the pitch continue.
Waves seem spreading form the body center to the perimeter. High pressure gives way to low pressure at latter half and then recovers.
Many tones seem taking a '8-shape' orbit as their main route in high/low pressure in the cello body like a favorite highway.
The length of the '8-shape' orbit is equivalent to the wavelength, the round time equals to the tone period.
The driving force of this mechanism is the speed of sound(340mm per 1mS). Cello body confines its resonance inside the (small) body controlling all within 2-4mS.
All high/low pressure air particles seem combined by strings of sound speed like the gravitation. This interesting mechanism might be the second invention of the violin family instrument.
Details will be reported in 2023.
Celloの最低音は C(66Hz,周期15.2mS,波長5.2m)であり、A線開放弦は A(221Hz,4.5mS,波長1.5m)である。
CelloのPizzicatoの先頭の波形を丁寧に観察していて気付くことがある。
共通するのは、最初に短い約4mSの期間があり、その後は各々のピッチが各々の周期で整然と繰り返されることである。
最初の4mSにおいて何が行われるのかは不明であるが、この時間はどの振動でもチェロ筐体内を1往復して元戻りできる時間に等しい。
この期間に各々1回の高密度と低密度が経験され、以後の連続した共鳴に対しての準備を行っているかのように見える。
定常的な共鳴が始まると 一般的には、高気圧が中心部分から周辺へ広がり、前半が全体高密度、後半が全体低密度に変化する。
その中で中心となる伝播経路は8の字形のコースをとることが多く両側に向けて8の字を描きながら1周する。
この8の字のトンネルの1周長はそのピッチの波長に等しく周回時間は周期に等しい。
筐体内でこれらのメカニズムを動かし結び付けているのは「音速(340mm/mS)」である。音速が万有引力のようにあらゆる点を結び付けているように見える。
チェロの長波長・長周期の共鳴を8の字形の小筐体を使ってタイムリーに制御しているといえる。
この魔法のような機能、これこそがCELLO(Violin属)の筐体に秘められた第2の偉大な発明なのかもしれない。
詳細は 2023年 報告予定。